problem solving in probability and statistics

Probability Questions with Solutions

Tutorial on finding the probability of an event. In what follows, S is the sample space of the experiment in question and E is the event of interest. n(S) is the number of elements in the sample space S and n(E) is the number of elements in the event E.

Questions and their Solutions

Answers to the above exercises, more references and links.

Statistics and Probability Worksheets

Welcome to the statistics and probability page at Math-Drills.com where there is a 100% chance of learning something! This page includes Statistics worksheets including collecting and organizing data, measures of central tendency (mean, median, mode and range) and probability.

Students spend their lives collecting, organizing, and analyzing data, so why not teach them a few skills to help them on their way. Data management is probably best done on authentic tasks that will engage students in their own learning. They can collect their own data on topics that interest them. For example, have you ever wondered if everyone shares the same taste in music as you? Perhaps a survey, a couple of graphs and a few analysis sentences will give you an idea.

Statistics has applications in many different fields of study. Budding scientists, stock market brokers, marketing geniuses, and many other pursuits will involve managing data on a daily basis. Teaching students critical thinking skills related to analyzing data they are presented will enable them to make crucial and informed decisions throughout their lives.

Probability is a topic in math that crosses over to several other skills such as decimals, percents, multiplication, division, fractions, etc. Probability worksheets will help students to practice all of these skills with a chance of success!

Most Popular Statistics and Probability Worksheets this Week

Mean, Median, Mode and Range Worksheets

Calculating the mean, median, mode and range are staples of the upper elementary math curriculum. Here you will find worksheets for practicing the calculation of mean, median, mode and range. In case you're not familiar with these concepts, here is how to calculate each one. To calculate the mean, add all of the numbers in the set together and divide that sum by the number of numbers in the set. To calculate the median, first arrange the numbers in order, then locate the middle number. In sets where there are an even number of numbers, calculate the mean of the two middle numbers. To calculate the mode, look for numbers that repeat. If there is only one of each number, the set has no mode. If there are doubles of two different numbers and there are more numbers in the set, the set has two modes. If there are triples of three different numbers and there are more numbers in the set, the set has three modes, and so on. The range is calculated by subtracting the least number from the greatest number.

Note that all of the measures of central tendency are included on each page, but you don't need to assign them all if you aren't working on them all. If you're only working on mean, only assign students to calculate the mean.

In order to determine the median, it is necessary to have your numbers sorted. It is also helpful in determining the mode and range. To expedite the process, these first worksheets include the lists of numbers already sorted.

• Calculating Mean, Median, Mode and Range from Sorted Lists Sets of 5 Numbers from 1 to 10 Sets of 5 Numbers from 10 to 99 Sets of 5 Numbers from 100 to 999 Sets of 10 Numbers from 1 to 10 Sets of 10 Numbers from 10 to 99 Sets of 10 Numbers from 100 to 999 Sets of 20 Numbers from 10 to 99 Sets of 15 Numbers from 100 to 999

Normally, data does not come in a sorted list, so these worksheets are a little more realistic. To find some of the statistics, it will be easier for students to put the numbers in order first.

• Calculating Mean, Median, Mode and Range from Unsorted Lists Sets of 5 Numbers from 1 to 10 Sets of 5 Numbers from 10 to 99 Sets of 5 Numbers from 100 to 999 Sets of 10 Numbers from 1 to 10 Sets of 10 Numbers from 10 to 99 Sets of 10 Numbers from 100 to 999 Sets of 20 Numbers from 10 to 99 Sets of 15 Numbers from 100 to 999

Collecting and Organizing Data

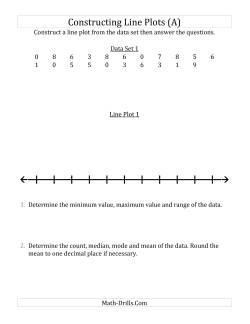
Teaching students how to collect and organize data enables them to develop skills that will enable them to study topics in statistics with more confidence and deeper understanding.

• Constructing Line Plots from Small Data Sets Construct Line Plots with Smaller Numbers and Lines with Ticks Provided (Small Data Set) Construct Line Plots with Smaller Numbers and Lines Only Provided (Small Data Set) Construct Line Plots with Smaller Numbers (Small Data Set) Construct Line Plots with Larger Numbers and Lines with Ticks Provided (Small Data Set) Construct Line Plots with Larger Numbers and Lines Only Provided (Small Data Set) Construct Line Plots with Larger Numbers (Small Data Set)
• Constructing Line Plots from Larger Data Sets Construct Line Plots with Smaller Numbers and Lines with Ticks Provided Construct Line Plots with Smaller Numbers and Lines Only Provided Construct Line Plots with Smaller Numbers Construct Line Plots with Larger Numbers and Lines with Ticks Provided Construct Line Plots with Larger Numbers and Lines Only Provided Construct Line Plots with Larger Numbers

Interpreting and Analyzing Data

Answering questions about graphs and other data helps students build critical thinking skills. Standard questions include determining the minimum, maximum, range, count, median, mode, and mean.

• Answering Questions About Stem-and-Leaf Plots Stem-and-Leaf Plots with about 25 data points Stem-and-Leaf Plots with about 50 data points Stem-and-Leaf Plots with about 100 data points
• Answering Questions About Line Plots Line Plots with Smaller Data Sets and Smaller Numbers Line Plots with Smaller Data Sets and Larger Numbers Line Plots with Larger Data Sets and Smaller Numbers Line Plots with Larger Data Sets and Larger Numbers
• Answering Questions About Broken-Line Graphs Answer Questions About Broken-Line Graphs
• Answering Questions About Circle Graphs Circle Graph Questions (Color Version) Circle Graph Questions (Black and White Version) Circle Graphs No Questions (Color Version) Circle Graphs No Questions (Black and White Version)
• Answering Questions About Pictographs Answer Questions About Pictographs

Probability Worksheets

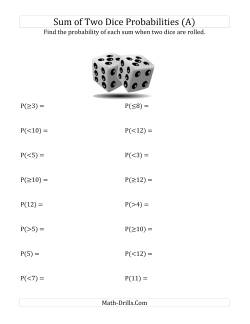
• Calculating Probabilities with Dice Sum of Two Dice Probabilities Sum of Two Dice Probabilities (with table)

Spinners can be used for probability experiments or for theoretical probability. Students should intuitively know that a number that is more common on a spinner will come up more often. Spinning 100 or more times and tallying the results should get them close to the theoretical probability. The more sections there are, the more spins will be needed.

• Calculating Probabilities with Number Spinners Number Spinner Probability (4 Sections) Number Spinner Probability (5 Sections) Number Spinner Probability (6 Sections) Number Spinner Probability (7 Sections) Number Spinner Probability (8 Sections) Number Spinner Probability (9 Sections) Number Spinner Probability (10 Sections) Number Spinner Probability (11 Sections) Number Spinner Probability (12 Sections)

Non-numerical spinners can be used for experimental or theoretical probability. There are basic questions on every version with a couple extra questions on the A and B versions. Teachers and students can make up other questions to ask and conduct experiments or calculate the theoretical probability. Print copies for everyone or display on an interactive white board.

• Probability with Single-Event Spinners Animal Spinner Probability ( 4 Sections) Animal Spinner Probability ( 5 Sections) Animal Spinner Probability ( 10 Sections) Letter Spinner Probability ( 4 Sections) Letter Spinner Probability ( 5 Sections) Letter Spinner Probability ( 10 Sections) Color Spinner Probability ( 4 Sections) Color Spinner Probability ( 5 Sections) Color Spinner Probability ( 10 Sections)
• Probability with Multi-Event Spinners Animal/Letter Combined Spinner Probability ( 4 Sections) Animal/Letter Combined Spinner Probability ( 5 Sections) Animal/Letter Combined Spinner Probability ( 10 Sections) Animal/Letter/Color Combined Spinner Probability ( 4 Sections) Animal/Letter/Color Combined Spinner Probability ( 5 Sections) Animal/Letter/Color Combined Spinner Probability ( 10 Sections)

Copyright © 2005-2024 Math-Drills.com You may use the math worksheets on this website according to our Terms of Use to help students learn math.

High Impact Tutoring Built By Math Experts

Personalized standards-aligned one-on-one math tutoring for schools and districts

Free ready-to-use math resources

Hundreds of free math resources created by experienced math teachers to save time, build engagement and accelerate growth

15 Probability Questions And Practice Problems for Middle and High School: Harder Exam Style Questions Included

Beki Christian

Probability questions and probability problems require students to work out how likely it is that something is to happen. Probabilities can be described using words or numbers. Probabilities range from 0 to 1 and can be written as fractions, decimals or percentages .

Here you’ll find a selection of probability questions of varying difficulty showing the variety you are likely to encounter in middle school and high school, including several harder exam style questions.

What are some real life examples of probability?

The more likely something is to happen, the higher its probability. We think about probabilities all the time.

For example, you may have seen that there is a 20% chance of rain on a certain day or thought about how likely you are to roll a 6 when playing a game, or to win in a raffle when you buy a ticket.

Probability Questions For Middle & High School

Get all the questions from this blog in an easy-to-download format, including answer key. Includes a mix of worded problems and deeper problem solving questions.

How to calculate probabilities

The probability of something happening is given by:

We can also use the following formula to help us calculate probabilities and solve problems:

• Probability of something not occuring = 1 – probability of if occurring P(not\;A) = 1 - P(A)
• For mutually exclusive events: Probability of event A OR event B occurring = Probability of event A + Probability of event B P(A\;or\;B) = P(A)+P(B)
• For independent events: Probability of event A AND event B occurring = Probability of event A times probability of event B P(A\;and\;B) = P(A) × P(B)

Probability question: A worked example

Question: What is the probability of getting heads three times in a row when flipping a coin?

When flipping a coin, there are two possible outcomes – heads or tails. Each of these options has the same probability of occurring during each flip. The probability of either heads or tails on a single coin flip is ½.

Since there are only two possible outcomes and they have the same probability of occurring, this is called a binomial distribution.

Let’s look at the possible outcomes if we flipped a coin three times.

Let H=heads and T=tails.

The possible outcomes are: HHH, THH, THT, HTT, HHT, HTH, TTH, TTT

Each of these outcomes has a probability of ⅛.

Therefore, the probability of flipping a coin three times in a row and having it land on heads all three times is ⅛.

Middle school probability questions

In middle school, probability questions introduce the idea of the probability scale and the fact that probabilities sum to one. We look at theoretical and experimental probability as well as learning about sample space diagrams and venn diagrams.

6th grade probability questions

1. Which number could be added to this spinner to make it more likely that the spinner will land on an odd number than a prime number?

Currently there are two odd numbers and two prime numbers so the chances of landing on an odd number or a prime number are the same. By adding 3, 5 or 11 you would be adding one prime number and one odd number so the chances would remain equal.

By adding 9 you would be adding an odd number but not a prime number. There would be three odd numbers and two prime numbers so the spinner would be more likely to land on an odd number than a prime number.

2. Ifan rolls a fair dice, with sides labeled A, B, C, D, E and F. What is the probability that the dice lands on a vowel?

A and E are vowels so there are 2 outcomes that are vowels out of 6 outcomes altogether.

Therefore the probability is   \frac{2}{6} which can be simplified to \frac{1}{3} .

7th grade probability questions

3. Max tested a coin to see whether it was fair. The table shows the results of his coin toss experiment:

Heads          Tails

    26                  41

What is the relative frequency of the coin landing on heads?

Max tossed the coin 67 times and it landed on heads 26 times.

\text{Relative frequency (experimental probability) } = \frac{\text{number of successful trials}}{\text{total number of trials}} = \frac{26}{67}

4. Grace rolled two dice. She then did something with the two numbers shown. Here is a sample space diagram showing all the possible outcomes:

What did Grace do with the two numbers shown on the dice?

Add them together

Subtract the number on dice 2 from the number on dice 1

Multiply them

Subtract the smaller number from the bigger number

For each pair of numbers, Grace subtracted the smaller number from the bigger number.

For example, if she rolled a 2 and a 5, she did 5 − 2 = 3.

8th grade probability questions

5. Alice has some red balls and some black balls in a bag. Altogether she has 25 balls. Alice picks one ball from the bag. The probability that Alice picks a red ball is x and the probability that Alice picks a black ball is 4x. Work out how many black balls are in the bag.

Since the probability of mutually exclusive events add to 1:  

\begin{aligned} x+4x&=1\\\\ 5x&=1\\\\ x&=\frac{1}{5} \end{aligned}

\frac{1}{5} of the balls are red and \frac{4}{5} of the balls are blue.

6. Arthur asked the students in his class whether they like math and whether they like science. He recorded his results in the venn diagram below.

How many students don’t like science?

We need to look at the numbers that are not in the ‘Like science’ circle. In this case it is 9 + 7 = 16.

High school probability questions

In high school, probability questions involve more problem solving to make predictions about the probability of an event. We also learn about probability tree diagrams, which can be used to represent multiple events, and conditional probability.

9th grade probability questions

7. A restaurant offers the following options:

Starter – soup or salad

Main – chicken, fish or vegetarian

Dessert – ice cream or cake

How many possible different combinations of starter, main and dessert are there?

The number of different combinations is 2 × 3 × 2 = 12.

8. There are 18 girls and 12 boys in a class. \frac{2}{9} of the girls and \frac{1}{4} of the boys walk to school. One of the students who walks to school is chosen at random. Find the probability that the student is a boy. 

First we need to work out how many students walk to school:

\frac{2}{9} \text{ of } 18 = 4

\frac{1}{4} \text{ of } 12 = 3

7 students walk to school. 4 are girls and 3 are boys. So the probability the student is a boy is \frac{3}{7} .

9. Rachel flips a biased coin. The probability that she gets two heads is 0.16. What is the probability that she gets two tails?

We have been given the probability of getting two heads. We need to calculate the probability of getting a head on each flip.

Let’s call the probability of getting a head p.

The probability p, of getting a head AND getting another head is 0.16.

Therefore to find p:

The probability of getting a head is 0.4 so the probability of getting a tail is 0.6.

The probability of getting two tails is 0.6 × 0.6 = 0.36 .

10th grade probability questions

10. I have a big tub of jelly beans. The probability of picking each different color of jelly bean is shown below:

If I were to pick 60 jelly beans from the tub, how many orange jelly beans would I expect to pick?

First we need to calculate the probability of picking an orange. Probabilities sum to 1 so 1 − (0.2 + 0.15 + 0.1 + 0.3) = 0.25.

The probability of picking an orange is 0.25.

The number of times I would expect to pick an orange jelly bean is 0.25 × 60 = 15 .

11. Dexter runs a game at a fair. To play the game, you must roll a dice and pick a card from a deck of cards.

To win the game you must roll an odd number and pick a picture card. The game can be represented by the tree diagram below.

Dexter charges players $1 to play and gives $3 to any winners. If 260 people play the game, how much profit would Dexter expect to make?

Completing the tree diagram:

Probability of winning is \frac{1}{2} \times \frac{4}{13} = \frac{4}{26}

If 260 play the game, Dexter would receive $260.

The expected number of winners would be \frac{4}{26} \times 260 = 40

Dexter would need to give away 40 × $3 = $120 .

Therefore Dexter’s profit would be $260 − $120 = $140.

12. A fair coin is tossed three times. Work out the probability of getting two heads and one tail.

There are three ways of getting two heads and one tail: HHT, HTH or THH.

The probability of each is \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2} = \frac{1}{8} 

Therefore the total probability is \frac{1}{8} +\frac{1}{8} + \frac{1}{8} = \frac{3}{8}

11th/12th grade probability questions

13. 200 people were asked about which athletic event they thought was the most exciting to watch. The results are shown in the table below.

A person is chosen at random. Given that that person chose 100m, what is the probability that the person was female?

Since we know that the person chose 100m, we need to include the people in that column only.

In total 88 people chose 100m so the probability the person was female is \frac{32}{88}   .

14.   Sam asked 50 people whether they like vegetable pizza or pepperoni pizza.

37 people like vegetable pizza. 

25 people like both. 

3 people like neither.

Sam picked one of the 50 people at random. Given that the person he chose likes pepperoni pizza, find the probability that they don’t like vegetable pizza.

We need to draw a venn diagram to work this out.

We start by putting the 25 who like both in the middle section. The 37 people who like vegetable pizza includes the 25 who like both, so 12 more people must like vegetable pizza. 3 don’t like either. We have 50 – 12 – 25 – 3 = 10 people left so this is the number that must like only pepperoni.

There are 35 people altogether who like pepperoni pizza. Of these, 10 do not like vegetable pizza. The probability is   \frac{10}{35} .

15. There are 12 marbles in a bag. There are n red marbles and the rest are blue marbles. Nico takes 2 marbles from the bag. Write an expression involving n for the probability that Nico takes one red marble and one blue marble.

We need to think about this using a tree diagram. If there are 12 marbles altogether and n are red then 12-n are blue.

To get one red and one blue, Nico could choose red then blue or blue then red so the probability is:

Looking for more middle school and high school probability math questions?

• Ratio questions
• Algebra questions
• Trigonometry questions
• Venn diagram questions
• Long division questions
• Pythagorean theorem questions

Do you have students who need extra support in math? Give your students more opportunities to consolidate learning and practice skills through personalized math tutoring with their own dedicated online math tutor. Each student receives differentiated instruction designed to close their individual learning gaps, and scaffolded learning ensures every student learns at the right pace. Lessons are aligned with your state’s standards and assessments, plus you’ll receive regular reports every step of the way. Personalized one-on-one math tutoring programs are available for: – 2nd grade tutoring – 3rd grade tutoring – 4th grade tutoring – 5th grade tutoring – 6th grade tutoring – 7th grade tutoring – 8th grade tutoring Why not learn more about how it works ?

The content in this article was originally written by secondary teacher Beki Christian and has since been revised and adapted for US schools by elementary math teacher Katie Keeton.

Related articles

36 Math Problems For 1st Graders With Answers & Teaching Ideas

30 8th Grade Math Problems: Answers With Worked Examples

28 Math Problems For 2nd Graders With Answers & Teaching Ideas

37 Math Problems For 3rd Graders: Answers With Worked Examples

3rd to 8th Grade Practice Tests

Get ready for your state math test with our 3rd to 8th grade practice assessments. These 6 multiple-choice tests, created by US math experts, cover essential topics and include detailed answers for effective test prep. Aligned with Common Core Standards, they’re the perfect tool to build student confidence.

Privacy Overview

Browse Course Material

Course info, instructors.

• Dr. Jeremy Orloff
• Dr. Jennifer French Kamrin

Departments

• Mathematics

As Taught In

• Discrete Mathematics
• Probability and Statistics

Learning Resource Types

Introduction to probability and statistics, course description.

This course provides an elementary introduction to probability and statistics with applications. Topics include basic combinatorics, random variables, probability distributions, Bayesian inference, hypothesis testing, confidence intervals, and linear regression.

These same course materials, including interactive …

These same course materials, including interactive components (online reading questions and problem checkers) are available on MIT’s  Open Learning Library , which is free to use. You have the option to enroll and track your progress, or you can view and use the materials without enrolling.

You are leaving MIT OpenCourseWare

Teach yourself statistics

Probability Tutorial

Applied researchers make decisions under uncertainty. Probability theory makes it possible for researchers to quantify the extent of uncertainty inherent in their conclusions and inferences.

About the Tutorial

This tutorial covers the essence of probabilty theory clearly and simply in just a few short lessons. The tutorial focuses on six topics:

• Probability basics. To solve probability problems, it helps to know about sets, subsets, and statistical experiments.
• Probability problems. To solve probability problems, you need to understand the rules of probability; and you need to know how to count data points.
• Poker probability. To compute probabilities for poker hands, you rely on fundamental principles in probability. It's a great way to build analytical skill, and it's fun.
• Random variables. Random variables are at the heart of probability. They can be characterized by central tendency, variability, and a probability distribution.
• Discrete probability distributions. How to work with binomial, hypergeometric, multinomial, negative binomial, and Poisson distributions.
• Continuous probability distributions. How to work with normal, standard normal, chi-square, t-, and f-distributions.

How to Use This Tutorial

This tutorial is designed for students and researchers who have some familiarity with introductory statistics (e.g., a high school statistics course or Advanced Placement Statistics ). Even if you have no prior knowledge of probability, you can work through the tutorial at your own pace and teach yourself to solve common probability problems!

Individual lessons are accessible through the table of contents, which can be found in the vertical column on the left side of the page. You should work through lessons in the order in which they appear; because some lessons build on previous lessons.

Individual lessons are accessible through the table of contents, which can be accessed by tapping the "Probability: Table of Contents" button at the top of the page. You should work through lessons in the order in which they appear; because some lessons build on previous lessons.

Additional Helpful Resources

As you progress through the tutorial, take advantage of the following helpful resources.

• Analytical tools . Stat Trek provides a variety of analytical tools - online statistical tables, calculators, problem solvers - to take the drudgery out of computations. The tutorial will alert you to these tools, all of which are free.
• Sample problems . Most of the lessons include sample problems. The sample problems help you test your knowledge. They also illustrate shortcuts and solutions to common statistics problems.
• Jargon interpreter . Stat Trek's online Statistics Dictionary takes the mystery out of statistical jargon. If any term or concept is unclear, visit the dictionary for additional explanation.

Note : The dictionary can be accessed by clicking the Help tab in the main menu (located at the top of this web page).

Probability and Statistics for Deep Learning

Deep Learning is often called “Statistical Learning” and approached by many experts as statistical theory of the problem to find the best model approximation from a given collection of data. These models are produced today by Generative Deep Learning, which is a key to unlocking the latest sophisticated form of AI, comparable to human’s form of intelligence. AI is already present in our everyday lives, for example face recognition at airports, background generation in video conferencing, etc. You cannot understand CHAT GPT and Large LLMs if you don’t understand generative modeling and deep learning. This course will introduce important concepts of probability theory and statistics which are the foundation of today’s Deep Learning. It will cover many important algorithms and modelling used in discriminative and generative deep learning models. In addition, the course will introduce tools and underlying mathematical concepts of data interpretation that work with specific models of deep neural networks. Upon completion of this course, you will have acquired the background in probability and statistics necessary for Machine Learning, and have the ability to use TensorFlow to create and train neural networks for specific practical problems.   Course Highlights:

• Knowledge of probability theory basic rules: conditional probability, joint probabilities, marginal probability
• Quantify events: Random Variables and becoming familiar with representing big data
• Important Probabilistic Distributions for Deep Learning
• Important Statistical Learning concepts: Entropy, KL divergence
• Statistical Estimators: MLE and MAP
• Logistic Regression and probabilistic models for classification (Kernels)
• Bayesian concept learning
• Supervised Learning Algorithms: Decision Trees and Random Forest
• Unsupervised Learning Algorithms: K-Means & Gaussian models (mixture model)
• Structured Probabilistic Models: Probabilistic Graphical Model, Markov Model
• Generative modeling: what are the properties of good generative model,  (transformers, LLMs, Multimodal)
• Utilize TensorFlow 2.9 or PyTorch for real world example

Software:  TensorFlow 2.9 Probability, PyTorch, Python 3.9. We will use tools which are opensource and you will run it on UCSD Extension server using virtual environment setup for you with Jupyter notebook.    Course typically offered:  Online during our  Spring  and  Fall  academic quarters. Prerequisites:  Basic knowledge of Linear Algebra - concept of vectors and matrices. Next steps:  Upon completion, consider additional coursework in our specialized certificate in  Machine Learning Methods  to continue learning. More information:  For more information about this course, please contact  [email protected] .  

Course Information

Get more information.

• 858-534-3229
• [email protected]

This course applies to these programs

Course sessions, section id:, class type:.

No textbook required.

• No refunds after: 9/30/2024

Instructor: Biljana Aleksic

Related courses.

Discrete Math: Problem Solving for Engineering, Programming, & Science

Introduction to statistics, introduction to statistics using r.