problem solving dividing decimals

Dividing Decimals

How do we divide when there are decimal points involved?

Well, it is easier to divide by a whole number ... so multiply by 10 until it is!

Example: 15 divided by 0.2

When we multiply the 0.2 by 10 we get a whole number:

0.2 × 10 = 2

But we must also do it to the 15:

15 × 10 = 150

So 15 ÷ 0.2 has become 150 ÷ 2 ( both numbers are 10 times larger):

150 ÷ 2 = 75

And so the answer is:

15 ÷ 0.2 = 75

To divide decimal numbers:

Multiply the divisor by as many 10's as we need, until it is a whole number. Remember to multiply the dividend by the same number of 10's.

Multiplying by 10 is easy, we just shift one space over like this:

Example: Divide 6.4 by 0.4

Let us move one space for both :

6.4 0.4 is exactly the same as 64 4 as we did the move for both numbers .

Now we can calculate:

So the answer is:

6.4 0.4 = 16

Are there really 16 lots of 0.4 in 6.4 ? Let's see:

For harder questions we may need to use Long Division :

Example: Divide 0.539 by 0.11

First we need to make the move twice to make 0.11 into a whole number:

0.539 0.11 is exactly the same as 53.9 11

But what about 53.9? It still has a decimal point.

Well, we can ignore the decimal point in the dividend so long as we remember to put it back later .

First we do the calculation without the decimal point:

Now put the decimal point in the answer directly above the decimal point in the dividend:

The answer is 4.9

Another example:

Example: Divide 9.1 by 7

The divisor (7) is already a whole number, so no need for any moves.

Now, ignore the decimal point in the dividend and use Long Division:

Put the decimal point in the answer directly above the decimal point in the dividend:

The answer is 1.3

Have a look at these Decimal Division Animations for further help.

As a final check we can put our "common sense" hat on and think "is that the right size?", because we don't want to pay ten times too much for anything, nor do we want to get only one-tenth of what we need!

Math Article

Dividing Decimals

Dividing decimals is similar to the division of whole numbers except for the decimals point. A similar division approach (like we do for whole numbers) is followed for decimals in maths. Before understanding the division of decimals, let’s recall what decimals and the meaning of decimal points are. In Mathematics, decimals are specific types of numbers, with a whole number and the fractional part separated by a decimal point. The dot that exists between the whole number and fractions part is called the decimal point. We can also perform various arithmetic operations on these numbers, such as addition, subtraction, multiplication and division .

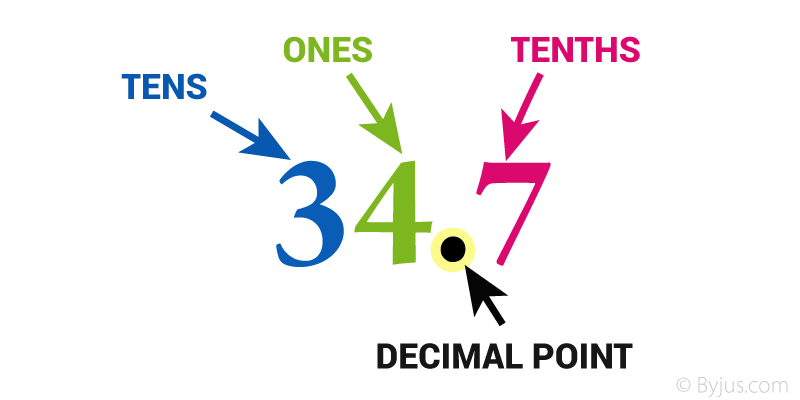
What is the Division of Decimals?

Dividing Decimals is similar to dividing whole numbers, except for the way we handle the decimal point. There are different cases in dividing decimals, such as:

Dividing Decimals by whole numbers

Division of a decimal number by another decimal number.

Division of decimals by 10, 100 and 1000

However, we may get a decimal or whole number when we divide decimal numbers. Let’s understand the division of these decimals in detail here.

There will be certain rules for dividing decimals in maths. These can be observed from the illustrative examples below.

The step wise procedure for dividing a decimal number by a whole number is given below for 1926 ÷ 4:

Step 1: Write the given division expression in the standard form.

Step 2: Now, we have to divide the decimal number’s whole number part by the divisor.

Step 3: Place the decimal point in the quotient above the dividend’s decimal point and then bring down the tenth digit.

Step 4: If the tenths digit of the obtained dividend cannot be divided by the divisor, write 0 in the quotient and in front of the tenths digit. Otherwise, proceed with the division process.

Step 5: Repeat the above step and keep adding the zeroes in the dividend until 0 is obtained in the remainder.

Therefore, 19.26 ÷ 4 = 4.815

That means, the quotient is 4.815 and the remainder is 0.

In this example, we considered only those divisions in which the number would be completely divisible by another number to give the remainder zero. However, there are some situations where the given decimal number may not be completely divisible by another number, i.e., we may not get a remainder zero. This can be understood from the example given below.

When we divide decimals, we have to convert the divisor to a whole number by moving the decimal point to the right. Then, we carry the dividend’s decimal point up to the same number of places to the right and divide the resultant numbers in the usual way as we perform in regular long division. This can be better understood with the help of the following example.

23.184 ÷ 0.3

The given Divisor = 0.3 and Dividend = 23.184

Change the divisor 0.3 to a whole number by moving the decimal point 1 place to the right. Then move the decimal point in the dividend the same, 1 place to the right.

23.184 ÷ 0.3 = 231.84 ÷ 3

Now, divide the decimal number 231.84 by the whole number., i.e. 3.

Dividing decimals by 10, 100 and 1000

Let us find the division of a decimal number by 10, 100 and 1000.

Consider 97.5 ÷ 10 = 9.75. Thus, the digits in 97.5 and 9.75 are the same, i.e., 9, 7, and 5, but the decimal point has shifted (towards left) in the quotient. Therefore, in a decimal division by 10, the decimal point will shift to the left by one place since 10 has only one zero over 1.

From the above example, we can say that in the division of decimals by 10, 100 or 1000, the number and quotient digits will be the same. However, the decimal point in the quotient shifts to the left by as many places as zeros over 1.

Dividing Decimals examples

The below solved examples help you in better understanding of the division of decimal numbers.

Example 1: Find the quotient for 1.764 ÷ 0.012.

The given Divisor = 0.012 and Dividend = 1.764

Now, change the divisor 0.012 to a whole number by moving the decimal point 3 places to the right. Then move the decimal point in the dividend the same, 3 places to the right.

1.764 ÷ 0.012 = 1764.0 ÷ 12

Thus, the quotient is 147.

Example 2: Calculate: 8.91 ÷ 2.2

The given Divisor = 2.2 and Dividend = 8.91

Now, change the divisor 2.2 to a whole number by moving the decimal point 1 place to the right. Then move the decimal point in the dividend the same, 1 place to the right.

8.91 ÷ 2.2 = 8910 ÷ 22

Dividing Decimals Problems

Solve the below practice problems on decimal division.

1. Divide 0.4545 by 3.6.

2. Find the remainder when 651.2 is divided by 0.04.

3. Evaluate: 7.75 ÷ 0.0025

Frequently Asked Questions – FAQs

How do you divide decimals step by step, which way do you move the decimal point when dividing by 10, how do you solve 5 divided by 100, what is 31 over 100 as a decimal, how do you divide a decimal by 10, leave a comment cancel reply.

Your Mobile number and Email id will not be published. Required fields are marked *

Request OTP on Voice Call

Post My Comment