the graphical representation of numerical data is called

• Maths Notes Class 9
• NCERT Solutions Class 9
• RD Sharma Solutions Class 9
• Maths Formulas Class 9
• Class 9 Syllabus
• Class 9 Revision Notes
• Physics Notes Class 9
• Chemistry Notes Class 9
• Biology Notes Class 9
• History Notes class 9
• Geography Notes class 9
• Social science Notes class 9

Graphical Representation of Data

Graphical Representation of Data: Graphical Representation of Data,” where numbers and facts become lively pictures and colorful diagrams . Instead of staring at boring lists of numbers, we use fun charts, cool graphs, and interesting visuals to understand information better. In this exciting concept of data visualization, we’ll learn about different kinds of graphs, charts, and pictures that help us see patterns and stories hidden in data.

There is an entire branch in mathematics dedicated to dealing with collecting, analyzing, interpreting, and presenting numerical data in visual form in such a way that it becomes easy to understand and the data becomes easy to compare as well, the branch is known as Statistics .

The branch is widely spread and has a plethora of real-life applications such as Business Analytics, demography, Astro statistics, and so on . In this article, we have provided everything about the graphical representation of data, including its types, rules, advantages, etc.

Table of Content

What is Graphical Representation

Types of graphical representations, line graphs, histograms , stem and leaf plot , box and whisker plot .

• Graphical Representations used in Maths

Value-Based or Time Series Graphs 

Frequency based, principles of graphical representations, advantages and disadvantages of using graphical system, general rules for graphical representation of data, frequency polygon, solved examples on graphical representation of data.

Graphics Representation is a way of representing any data in picturized form . It helps a reader to understand the large set of data very easily as it gives us various data patterns in visualized form.

There are two ways of representing data,

• Pictorial Representation through graphs.

They say, “A picture is worth a thousand words”.  It’s always better to represent data in a graphical format. Even in Practical Evidence and Surveys, scientists have found that the restoration and understanding of any information is better when it is available in the form of visuals as Human beings process data better in visual form than any other form.

Does it increase the ability 2 times or 3 times? The answer is it increases the Power of understanding 60,000 times for a normal Human being, the fact is amusing and true at the same time.

Check: Graph and its representations

Comparison between different items is best shown with graphs, it becomes easier to compare the crux of the data about different items. Let’s look at all the different types of graphical representations briefly: 

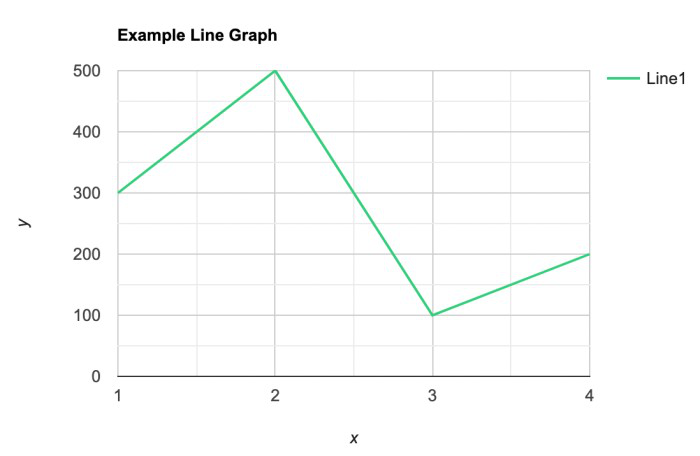
A line graph is used to show how the value of a particular variable changes with time. We plot this graph by connecting the points at different values of the variable. It can be useful for analyzing the trends in the data and predicting further trends. 

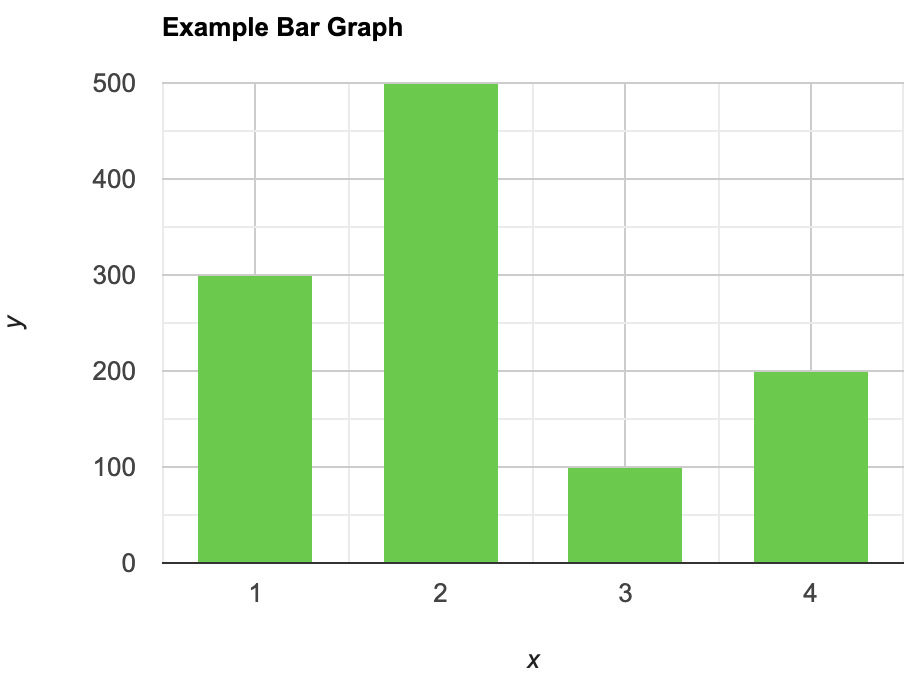
A bar graph is a type of graphical representation of the data in which bars of uniform width are drawn with equal spacing between them on one axis (x-axis usually), depicting the variable. The values of the variables are represented by the height of the bars. 

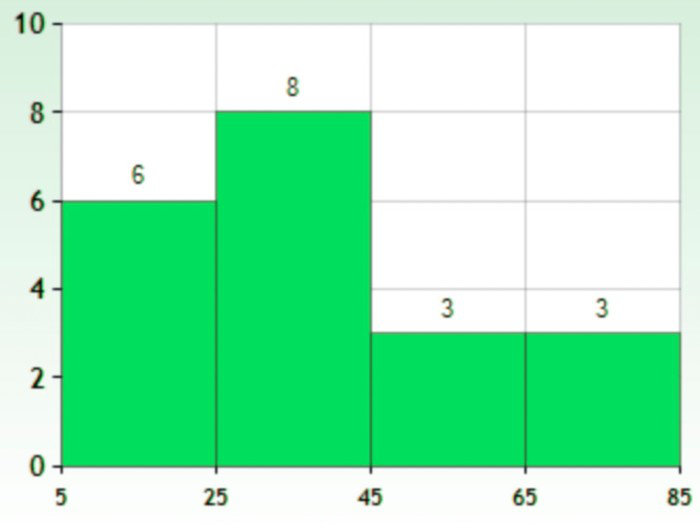
This is similar to bar graphs, but it is based frequency of numerical values rather than their actual values. The data is organized into intervals and the bars represent the frequency of the values in that range. That is, it counts how many values of the data lie in a particular range. 

It is a plot that displays data as points and checkmarks above a number line, showing the frequency of the point.  

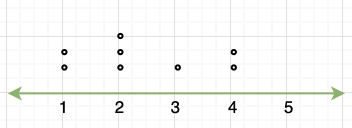
This is a type of plot in which each value is split into a “leaf”(in most cases, it is the last digit) and “stem”(the other remaining digits). For example: the number 42 is split into leaf (2) and stem (4).  

These plots divide the data into four parts to show their summary. They are more concerned about the spread, average, and median of the data. 

It is a type of graph which represents the data in form of a circular graph. The circle is divided such that each portion represents a proportion of the whole. 

Graphical Representations used in Math’s

Graphs in Math are used to study the relationships between two or more variables that are changing. Statistical data can be summarized in a better way using graphs. There are basically two lines of thoughts of making graphs in maths: 

• Value-Based or Time Series Graphs

These graphs allow us to study the change of a variable with respect to another variable within a given interval of time. The variables can be anything. Time Series graphs study the change of variable with time. They study the trends, periodic behavior, and patterns in the series. We are more concerned with the values of the variables here rather than the frequency of those values. 

Example: Line Graph

These kinds of graphs are more concerned with the distribution of data. How many values lie between a particular range of the variables, and which range has the maximum frequency of the values. They are used to judge a spread and average and sometimes median of a variable under study.

Also read: Types of Statistical Data

• All types of graphical representations follow algebraic principles.
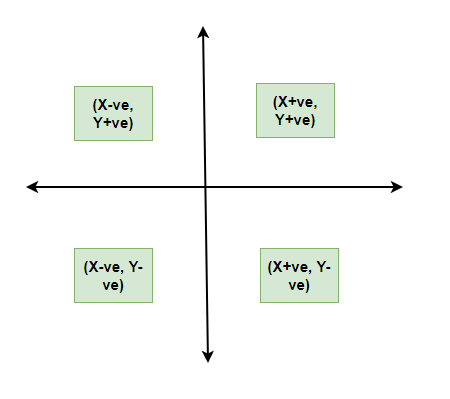
• When plotting a graph, there’s an origin and two axes.
• The x-axis is horizontal, and the y-axis is vertical.
• The axes divide the plane into four quadrants.
• The origin is where the axes intersect.
• Positive x-values are to the right of the origin; negative x-values are to the left.
• Positive y-values are above the x-axis; negative y-values are below.

• It gives us a summary of the data which is easier to look at and analyze.
• It saves time.
• We can compare and study more than one variable at a time.

Disadvantages

• It usually takes only one aspect of the data and ignores the other. For example, A bar graph does not represent the mean, median, and other statistics of the data. 
• Interpretation of graphs can vary based on individual perspectives, leading to subjective conclusions.
• Poorly constructed or misleading visuals can distort data interpretation and lead to incorrect conclusions.

Check : Diagrammatic and Graphic Presentation of Data

We should keep in mind some things while plotting and designing these graphs. The goal should be a better and clear picture of the data. Following things should be kept in mind while plotting the above graphs: 

• Whenever possible, the data source must be mentioned for the viewer.
• Always choose the proper colors and font sizes. They should be chosen to keep in mind that the graphs should look neat.
• The measurement Unit should be mentioned in the top right corner of the graph.
• The proper scale should be chosen while making the graph, it should be chosen such that the graph looks accurate.
• Last but not the least, a suitable title should be chosen.

A frequency polygon is a graph that is constructed by joining the midpoint of the intervals. The height of the interval or the bin represents the frequency of the values that lie in that interval. 

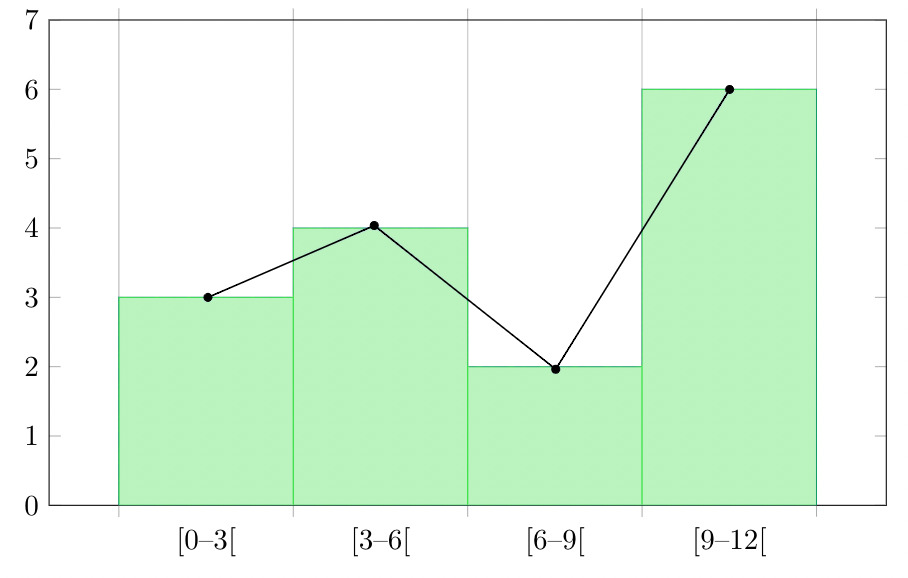
Question 1: What are different types of frequency-based plots? 

Types of frequency-based plots:  Histogram Frequency Polygon Box Plots

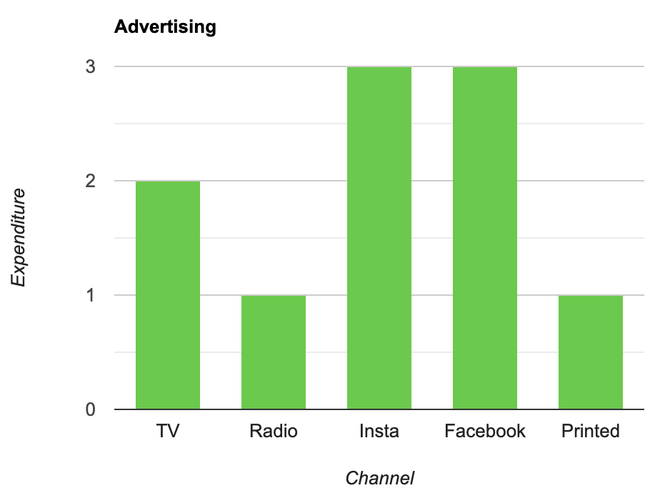
Question 2: A company with an advertising budget of Rs 10,00,00,000 has planned the following expenditure in the different advertising channels such as TV Advertisement, Radio, Facebook, Instagram, and Printed media. The table represents the money spent on different channels. 

Draw a bar graph for the following data. 

• Put each of the channels on the x-axis
• The height of the bars is decided by the value of each channel.

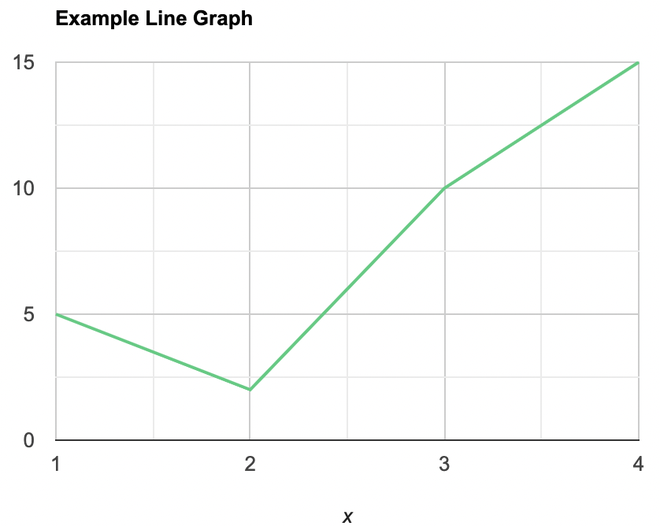
Question 3: Draw a line plot for the following data 

• Put each of the x-axis row value on the x-axis
• joint the value corresponding to the each value of the x-axis.

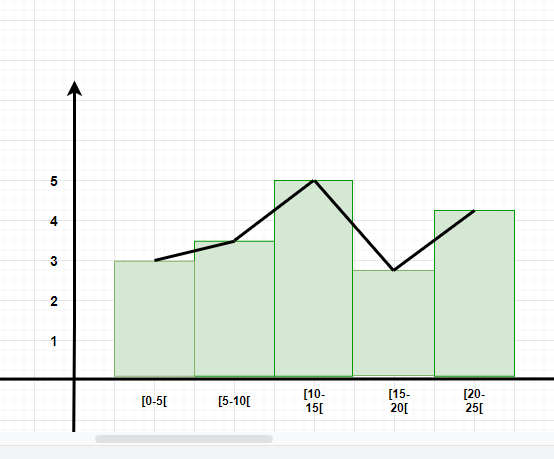
Question 4: Make a frequency plot of the following data: 

• Draw the class intervals on the x-axis and frequencies on the y-axis.
• Calculate the midpoint of each class interval.

Now join the mid points of the intervals and their corresponding frequencies on the graph. 

This graph shows both the histogram and frequency polygon for the given distribution.

Related Article:

Graphical Representation of Data| Practical Work in Geography Class 12 What are the different ways of Data Representation What are the different ways of Data Representation? Charts and Graphs for Data Visualization

Conclusion of Graphical Representation

Graphical representation is a powerful tool for understanding data, but it’s essential to be aware of its limitations. While graphs and charts can make information easier to grasp, they can also be subjective, complex, and potentially misleading . By using graphical representations wisely and critically, we can extract valuable insights from data, empowering us to make informed decisions with confidence.

Graphical Representation of Data – FAQs

What are the advantages of using graphs to represent data.

Graphs offer visualization, clarity, and easy comparison of data, aiding in outlier identification and predictive analysis.

What are the common types of graphs used for data representation?

Common graph types include bar, line, pie, histogram, and scatter plots , each suited for different data representations and analysis purposes.

How do you choose the most appropriate type of graph for your data?

Select a graph type based on data type, analysis objective, and audience familiarity to effectively convey information and insights.

How do you create effective labels and titles for graphs?

Use descriptive titles, clear axis labels with units, and legends to ensure the graph communicates information clearly and concisely.

How do you interpret graphs to extract meaningful insights from data?

Interpret graphs by examining trends, identifying outliers, comparing data across categories, and considering the broader context to draw meaningful insights and conclusions.

Similar Reads

• Mathematics
• School Learning
• Maths-Class-9

Improve your Coding Skills with Practice

What kind of Experience do you want to share?

Types of Graphs and Charts And Their Uses

If you are wondering what are the different types of graphs and charts ,   their uses and names, this page summarizes them with examples and pictures.

Although it is hard to tell what are all the types of graphs, this page consists all of the common types of statistical graphs and charts (and their meanings) widely used in any science.

1. Line Graphs

A line chart graphically displays data that changes continuously over time. Each line graph consists of points that connect data to show a trend (continuous change). Line graphs have an x-axis and a y-axis. In the most cases, time is distributed on the horizontal axis.

Uses of line graphs:

• When you want  to show trends . For example, how house prices have increased over time.
• When you want  to make predictions based on a data history over time.
• When comparing  two or more different variables, situations, and information over a given period of time.

The following line graph shows annual sales of a particular business company for the period of six consecutive years:

Note: the above example is with 1 line. However, one line chart can compare multiple trends by several distributing lines.

2. Bar Charts

Bar charts represent categorical data with rectangular bars (to understand what is categorical data see categorical data examples ). Bar graphs are among the most popular types of graphs and charts in economics, statistics, marketing, and visualization in digital customer experience . They are commonly used to compare several categories of data.

Each rectangular bar has length and height proportional to the values that they represent.

One axis of the bar chart presents the categories being compared. The other axis shows a measured value.

Bar Charts Uses:

• When you want to display data that are grouped into nominal or ordinal categories (see nominal vs ordinal data ).
• To compare data among different categories.
• Bar charts can also show large   data changes over time.
• Bar charts are ideal for visualizing the distribution of data when we have more than three categories.

The bar chart below represents the total sum of sales for Product A and Product B over three years.

The bars are 2 types: vertical or horizontal. It doesn’t matter which kind you will use. The above one is a vertical type.

3. Pie Charts

When it comes to statistical types of graphs and charts, the pie chart (or the circle chart) has a crucial place and meaning. It displays data and statistics in an easy-to-understand ‘pie-slice’ format and illustrates numerical proportion.

Each pie slice is relative to the size of a particular category in a given group as a whole. To say it in another way, the pie chart brakes down a group into smaller pieces. It shows part-whole relationships.

To make a pie chart, you need a list of categorical variables and numerical variables.

Pie Chart Uses:

• When you want to create and represent the composition of something.
• It is very useful for displaying nominal or ordinal categories of data.
• To show percentage or proportional data.
• When comparing areas of growth within a business such as profit.
• Pie charts work best for displaying data for 3 to 7 categories.

The pie chart below represents the proportion of types of transportation used by 1000 students to go to their school.

Pie charts are widely used by data-driven marketers for displaying marketing data.

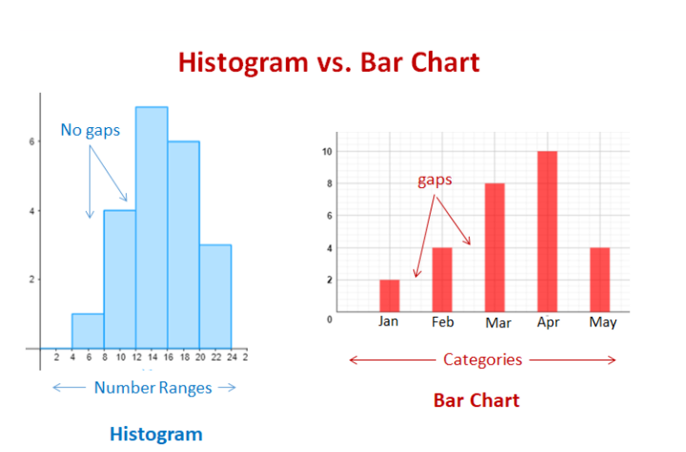
4. Histogram

A histogram shows continuous data in ordered rectangular columns (to understand what is continuous data see our post discrete vs continuous data ). Usually, there are no gaps between the columns.

The histogram displays a frequency distribution (shape) of a data set. At first glance, histograms look alike to bar graphs. However, there is a key difference between them. Bar Chart represents categorical data and histogram represent continuous data.

Histogram Uses:

• When the data is continuous .
• When you want to represent the shape of the data’s distribution .
• When you want to see whether the outputs of two or more processes are different.
• To summarize large data sets graphically.
• To communicate the data distribution quickly to others.

The histogram below represents per capita income for five age groups.

Histograms are very widely used in statistics, business, and economics.

5. Scatter plot

The scatter plot is an X-Y diagram that shows a relationship between two variables. It is used to plot data points on a vertical and a horizontal axis. The purpose is to show how much one variable affects another.

Usually, when there is a relationship between 2 variables, the first one is called independent. The second variable is called dependent because its values depend on the first variable.

Scatter plots also help you predict the behavior of one variable (dependent) based on the measure of the other variable (independent).

Scatter plot uses:

• When trying to find out whether there is a relationship between 2 variables .
• To predict  the behavior of dependent variable based on the measure of the independent variable.
• When having paired numerical data.
• When working with  root cause analysis tools  to identify the potential for problems.
• When you just want to visualize the correlation between 2 large datasets without regard to time .

The below Scatter plot presents data for 7 online stores, their monthly e-commerce sales, and online advertising costs for the last year.

The orange line you see in the plot is called “line of best fit” or a “trend line”. This line is used to help us make predictions that are based on past data.

The Scatter plots are used widely in data science and statistics. They are a great tool for visualizing linear regression models .

More examples and explanation for scatter plots you can see in our post what does a scatter plot show and simple linear regression examples .

6. Venn Chart

Venn Diagram (also called primary diagram, set diagram or logic diagrams) uses overlapping circles to visualize the logical relationships between two or more group of items.

Venn Diagram is one of the types of graphs and charts used in scientific and engineering presentations, in computer applications, in maths, and in statistics.

The basic structure of the Venn diagram is usually overlapping circles. The items in the overlapping section have specific common characteristics. Items in the outer portions of the circles do not have common traits.

Venn Chart Uses:

• When you want to compare and contrast groups of things.
• To categorize or group items.
• To illustrate logical relationships from various datasets.
• To identify all the possible relationships between collections of datasets.

The following science example of Venn diagram compares the features of birds and bats.

7. Area Charts 

Area Chart Uses:

• When you want to show trends , rather than express specific values.
• To show a simple comparison of the trend of data sets over the period of time.
• To display the magnitude of a change.
• To compare a small number of categories.

The area chart has 2 variants: a variant with data plots overlapping each other and a variant with data plots stacked on top of each other (known as stacked area chart – as the shown in the following example).

The area chart below shows quarterly sales for product categories A and B for the last year.

This area chart shows you a quick comparison of the trend in the quarterly sales of Product A and Product B over the period of the last year.

8. Spline Chart

The Spline Chart is one of the most widespread types of graphs and charts used in statistics. It is a form of the line chart that represent smooth curves through the different data points.

Spline charts possess all the characteristics of a line chart except that spline charts have a fitted curved line to join the data points. In comparison, line charts connect data points with straight lines.

Spline Chart   Uses:

• When you want to plot data that requires the usage of curve-fitting such as a product lifecycle chart or an impulse-response chart.
• Spline charts are often used in designing Pareto charts .
• Spline chart also is often used for data modeling by when you have limited number of data points and estimating the intervening values.

The following spline chart example shows sales of a company through several months of a year:

9. Box and Whisker Chart

A box and whisker chart is a statistical graph for displaying sets of numerical data through their quartiles. It displays a frequency distribution of the data.

The box and whisker chart helps you to display the spread and skewness for a given set of data using the five number summary principle: minimum, maximum, median, lower and upper quartiles. The ‘five-number summary’ principle allows providing a statistical summary for a particular set of numbers. It shows you the range (minimum and maximum numbers), the spread (upper and lower quartiles), and the center (median) for the set of data numbers.

A very simple figure of a box and whisker plot you can see below:

Box and Whisker Chart Uses:

• When you want to observe the upper, lower quartiles, mean, median, deviations, etc. for a large set of data.
• When you want to see a quick view of the dataset distribution .
• When you have multiple data sets that come from independent sources and relate to each other in some way.
• When you need to compare data from different categories.

The table and box-and-whisker plots below shows test scores for Maths and Literature for the same class.

Box and Whisker charts have applications in many scientific areas and types of analysis such as statistical analysis, test results analysis, marketing analysis, data analysis, and etc.

10. Bubble Chart

Bubble charts are super useful types of graphs for making a comparison of the relationships between data in 3 numeric-data dimensions: the Y-axis data, the X-axis data, and data depicting the bubble size.

Bubble charts are very similar to XY Scatter plots but the bubble chart adds more functionality – a third dimension of data that can be extremely valuable.

Both axes (X and Y) of a bubble chart are numeric.

Bubble Chart Uses:

• When you have to display three or four dimensions of data.
• When you want to compare and display the relationships between categorized circles, by the use of proportions.

The bubble chart below shows the relationship between Cost (X-Axis), Profit (Y-Axis), and Probability of Success (%) (Bubble Size).

11. Pictographs

The pictograph or a pictogram is one of the more visually appealing types of graphs and charts that display numerical information with the use of icons or picture symbols to represent data sets.

They are very easy to read statistical way of data visualization. A pictogram shows the frequency of data as images or symbols. Each image/symbol may represent one or more units of a given dataset.

Pictograph Uses:

• When your audience prefers and understands better displays that include icons and illustrations. Fun can promote learning.
• It’s habitual for infographics to use of a pictogram.
• When you want to compare two points  in an emotionally powerful way.

The following pictographic represents the number of computers sold by a business company for the period from January to March.

The pictographic example above shows that in January are sold 20 computers (4×5 = 20), in February are sold 30 computers (6×5 = 30) and in March are sold 15 computers.

12. Dot Plot

Dot plot or dot graph is just one of the many types of graphs and charts to organize statistical data. It uses dots to represent data. A Dot Plot is used for relatively small sets of data and the values fall into a number of discrete categories.

If a value appears more than one time, the dots are ordered one above the other. That way the column height of dots shows the frequency for that value.

Dot Plot Uses:

• To plot frequency counts when you have a small number of categories .
• Dot plots are very useful when the variable is quantitative or categorical .
• Dot graphs are also used for univariate data (data with only one variable that you can measure).

Suppose you have a class of 26 students. They are asked to tell their favorite color. The dot plot below represents their choices:

It is obvious that blue is the most preferred color by the students in this class.

13. Radar Chart

A radar chart is one of the most modern types of graphs and charts – ideal for multiple comparisons. Radar charts use a circular display with several different quantitative axes looking like spokes on a wheel. Each axis shows a quantity for a different categorical value.

Radar charts are also known as spider charts, web charts, star plots, irregular polygons, polar charts, cobweb charts or Kiviat diagram.

Radar Chart has many applications nowadays in statistics, maths, business, sports analysis, data intelligence, and etc.

Radar Chart Uses:

• When you want to observe which variables have similar values or whether there are any outliers amongst each variable.
• To represent  multiple comparisons .
• When you want to see which variables are scoring low or high within a dataset. This makes radar chart ideal for displaying performance .

For example, we can compare employee’s performance with the scale of 1-8 on subjects such as Punctuality, Problem-solving, Meeting Deadlines, Marketing Knowledge, Communications. A point that is closer to the center on an axis shows a lower value and a worse performance.

It is obvious that Jane has a better performance than Samanta.

14. Pyramid Graph

When it comes to easy to understand and good looking types of graphs and charts, pyramid graph has a top place.

A pyramid graph is a chart in a pyramid shape or triangle shape. These types of charts are best for data that is organized in some kind of hierarchy. The levels show a progressive order.

Pyramid Graph Uses:

• When you want to indicate a hierarchy level among the topics or other types of data.
• Pyramid graph is often used to represent progressive orders such as: “older to newer”, “more important to least important”, “specific to least specific”‘ and etc.
• When you have a proportional or interconnected relationship between data sets.

A classic pyramid graph example is the healthy food pyramid that shows fats, oils, and sugar (at the top) should be eaten less than many other foods such as vegetables and fruits (at the bottom of the pyramid).

Conclusion:

You might know that choosing the right type of chart is some kind of tricky business.

Anyway, you have a wide choice of types of graphs and charts. Used in the right way, they are a powerful weapon to help you make your reports and presentations both professional and clear.

What are your favorite types of graphs and charts? Share your thoughts on the field below.

About The Author

Silvia Valcheva

Silvia Valcheva is a digital marketer with over a decade of experience creating content for the tech industry. She has a strong passion for writing about emerging software and technologies such as big data, AI (Artificial Intelligence), IoT (Internet of Things), process automation, etc.

10 Comments

I have learned a lot from your presentation. Very informative

Nicely described different graphs, I learned a lot.

very useful. exiting

I love this. I learned a lot.

Very good representation of date. I would suggest an addition of “stem and leaf” diagrams.

I have only one thing to say and that is this is the best representation of every graphs and charts I have ever seen 😀

Very well described. Great learning article for beginners on Charts.

Really helpful thanks

Very Helpful text; Thanks Silvia Valcheva for your hard work

Leave a Reply Cancel Reply

This site uses Akismet to reduce spam. Learn how your comment data is processed .

• Math Article

Graphical Representation

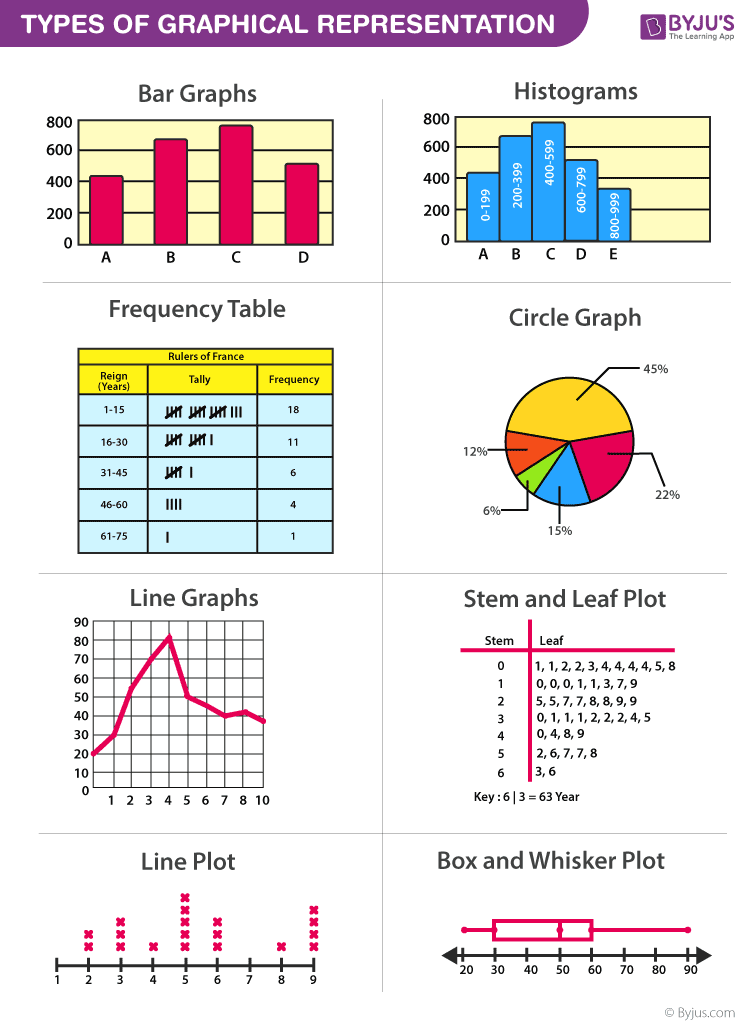
Graphical Representation is a way of analysing numerical data. It exhibits the relation between data, ideas, information and concepts in a diagram. It is easy to understand and it is one of the most important learning strategies. It always depends on the type of information in a particular domain. There are different types of graphical representation. Some of them are as follows:

• Line Graphs – Line graph or the linear graph is used to display the continuous data and it is useful for predicting future events over time.
• Bar Graphs – Bar Graph is used to display the category of data and it compares the data using solid bars to represent the quantities.
• Histograms – The graph that uses bars to represent the frequency of numerical data that are organised into intervals. Since all the intervals are equal and continuous, all the bars have the same width.
• Line Plot – It shows the frequency of data on a given number line. ‘ x ‘ is placed above a number line each time when that data occurs again.
• Frequency Table – The table shows the number of pieces of data that falls within the given interval.
• Circle Graph – Also known as the pie chart that shows the relationships of the parts of the whole. The circle is considered with 100% and the categories occupied is represented with that specific percentage like 15%, 56%, etc.
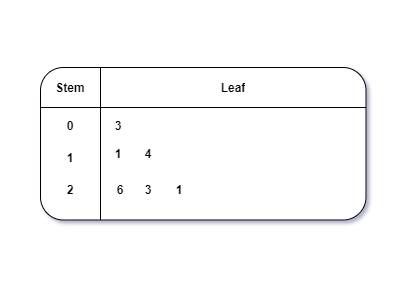
• Stem and Leaf Plot – In the stem and leaf plot, the data are organised from least value to the greatest value. The digits of the least place values from the leaves and the next place value digit forms the stems.
• Box and Whisker Plot – The plot diagram summarises the data by dividing into four parts. Box and whisker show the range (spread) and the middle ( median) of the data.

General Rules for Graphical Representation of Data

There are certain rules to effectively present the information in the graphical representation. They are:

• Suitable Title: Make sure that the appropriate title is given to the graph which indicates the subject of the presentation.
• Measurement Unit: Mention the measurement unit in the graph.
• Proper Scale: To represent the data in an accurate manner, choose a proper scale.
• Index: Index the appropriate colours, shades, lines, design in the graphs for better understanding.
• Data Sources: Include the source of information wherever it is necessary at the bottom of the graph.
• Keep it Simple: Construct a graph in an easy way that everyone can understand.
• Neat: Choose the correct size, fonts, colours etc in such a way that the graph should be a visual aid for the presentation of information.

Graphical Representation in Maths

In Mathematics, a graph is defined as a chart with statistical data, which are represented in the form of curves or lines drawn across the coordinate point plotted on its surface. It helps to study the relationship between two variables where it helps to measure the change in the variable amount with respect to another variable within a given interval of time. It helps to study the series distribution and frequency distribution for a given problem.  There are two types of graphs to visually depict the information. They are:

• Time Series Graphs – Example: Line Graph
• Frequency Distribution Graphs – Example: Frequency Polygon Graph

Principles of Graphical Representation

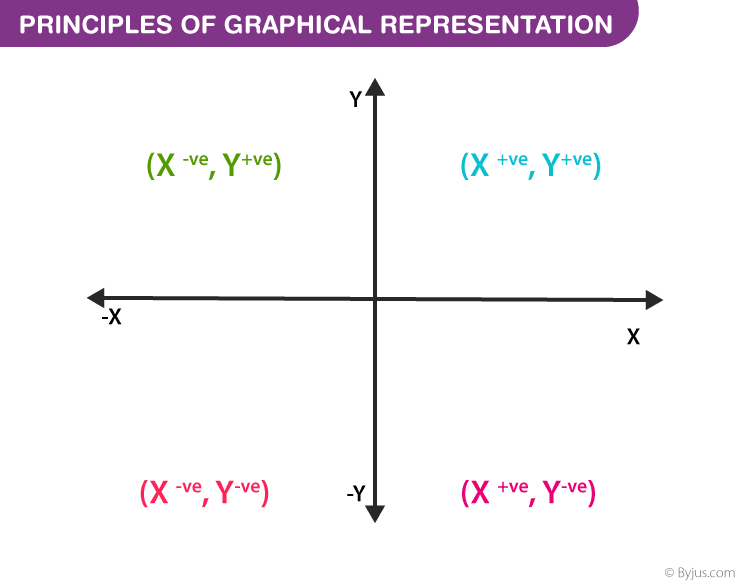
Algebraic principles are applied to all types of graphical representation of data. In graphs, it is represented using two lines called coordinate axes. The horizontal axis is denoted as the x-axis and the vertical axis is denoted as the y-axis. The point at which two lines intersect is called an origin ‘O’. Consider x-axis, the distance from the origin to the right side will take a positive value and the distance from the origin to the left side will take a negative value. Similarly, for the y-axis, the points above the origin will take a positive value, and the points below the origin will a negative value.

Generally, the frequency distribution is represented in four methods, namely

• Smoothed frequency graph
• Pie diagram
• Cumulative or ogive frequency graph
• Frequency Polygon

Merits of Using Graphs

Some of the merits of using graphs are as follows:

• The graph is easily understood by everyone without any prior knowledge.
• It saves time
• It allows us to relate and compare the data for different time periods
• It is used in statistics to determine the mean, median and mode for different data, as well as in the interpolation and the extrapolation of data.

Example for Frequency polygonGraph

Here are the steps to follow to find the frequency distribution of a frequency polygon and it is represented in a graphical way.

• Obtain the frequency distribution and find the midpoints of each class interval.
• Represent the midpoints along x-axis and frequencies along the y-axis.
• Plot the points corresponding to the frequency at each midpoint.
• Join these points, using lines in order.
• To complete the polygon, join the point at each end immediately to the lower or higher class marks on the x-axis.

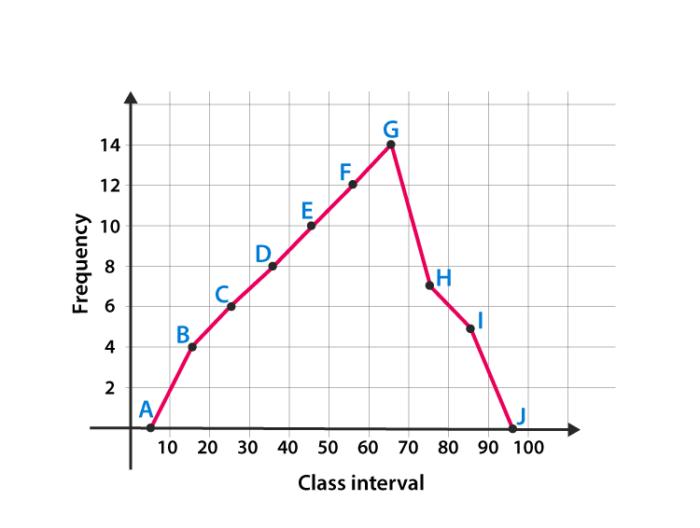
Draw the frequency polygon for the following data

Mark the class interval along x-axis and frequencies along the y-axis.

Let assume that class interval 0-10 with frequency zero and 90-100 with frequency zero.

Now calculate the midpoint of the class interval.

Using the midpoint and the frequency value from the above table, plot the points A (5, 0), B (15, 4), C (25, 6), D (35, 8), E (45, 10), F (55, 12), G (65, 14), H (75, 7), I (85, 5) and J (95, 0).

To obtain the frequency polygon ABCDEFGHIJ, draw the line segments AB, BC, CD, DE, EF, FG, GH, HI, IJ, and connect all the points.

Frequently Asked Questions

What are the different types of graphical representation.

Some of the various types of graphical representation include:

• Line Graphs
• Frequency Table
• Circle Graph, etc.

Read More:  Types of Graphs

What are the Advantages of Graphical Method?

Some of the advantages of graphical representation are:

• It makes data more easily understandable.
• It saves time.
• It makes the comparison of data more efficient.

Leave a Comment Cancel reply

Your Mobile number and Email id will not be published. Required fields are marked *

Request OTP on Voice Call

Post My Comment

Very useful for understand the basic concepts in simple and easy way. Its very useful to all students whether they are school students or college sudents

Thanks very much for the information

Register with BYJU'S & Download Free PDFs

Register with byju's & watch live videos.

• Increase Font Size

46 Presentation of data II – Graphical representation

Pa . Raajeswari

Graphical representation is the visual display of data using plots and charts. It is used in many academic and professional disciplines but most widely so in the fields of mathematics, medicine and sciences. Graphical representation helps to quantify, sort and present data in a method that is understandable to a large variety of audiences. A graph is the representation of data by using graphical symbols such as lines, bars, pie slices, dots etc. A graph does represent a numerical data in the form of a qualitative structure and provides important information.

Statistical surveys and experiments provides valuable information about numerical scores. For better understanding and making conclusions and interpretations, the data should be managed and organized in a systematic form.

Graphs also enable in studying both time series and frequency distribution as they give clear account and precise picture of problem. Above all graphs are also easy to understand and eye catching and can create a storing impact on memory.

General Principles of Graphic Representation:

There are some algebraic principles which apply to all types of graphic representation of data. In a graph there are two lines called coordinate axes. One is vertical known as Y axis and the other is horizontal called X axis. These two lines are perpendicular to each other. Where these two lines intersect each other is called ‘0’ or the Origin. On the X axis the distances right to the origin have positive value and distances left to the origin have negative value. On the Y axis distances above the origin have a positive value and below the origin have a negative value.

TYPES OF GRAPHICAL REPRESENTATON:

The various types of graphical representations of the data are

• Circle Graph
• Histogram and Frequency Polygon

1. Dot Plots

The dot plot is one of the most simplest ways of graphical representation of the statistical data. As the name itself suggests, a dot plot uses the dots. It is a graphic display which usually compares frequency within different categories. The dot plot is composed of dots that are to be plotted on a graph paper.

In the dot plot, every dot denotes a specific number of observations belonging to a data set. One dot usually represents one observation. These dots are to be marked in the form of a column for each category. In this way, the height of each column shows the corresponding frequency of some category. The dot plots are quite useful when there are small amount of data is given within the small number of categories.

2. Bar Graph

A bar graph is a very frequently used graph in statistics as well as in media. A bar graph is a type of graph which contains rectangles or rectangular bars. The lengths of these bars should be proportional to the numerical values represented by them. In bar graph, the bars may be plotted either horizontally or vertically. But a vertical bar graph (also known as column bar graph) is used more than a horizontal one.

A vertical bar graph is shown below:

Number of students went to different countries for study:

The rectangular bars are separated by some distance in order to distinguish them from one another. The bar graph shows comparison among the given categories.

Mostly, horizontal axis of the graph represents specific categories and vertical axis shows the discrete numerical values.

3.Line Graph

A line graph is a kind of graph which represents data in a way that a series of points are to be connected by segments of straight lines. In a line graph, the data points are plotted on a graph and they are joined together with straight line.

A   sample   line   graph   is    illustrated    in    the   following   diagram:

The line graphs are used in the science, statistics and media. Line graphs are very easy to create. These are quite popular in comparison with other graphs since they visualize characteristics revealing data trends very clearly. A line graph gives a clear visual comparison between two variables which are represented on X-axis and Y-axis.

4.Circle Graph

A circle graph is also known as a pie graph or pie chart. It is called so since it is similar to slice of a “pie”. A pie graph is defined as a graph which contains a circle which is divided into sectors. These sectors illustrate the numerical proportion of the data.

A pie chart are shown in the following diagram:

The arc lengths of the sectors, in pie chart, are proportional to the numerical value they represent.Circle graphs are quite commonly seen in mass media as well as in business world.

5. Histogram and Frequency Polygon

The histograms and frequency polygons are very common graphs in statistics. A histogram is defined as a graphical representation of the mutually exclusive events. A histogram is quite similar to the bar graph. Both are made up of rectangular bars. The difference is that there is no gap between any two bars in the histogram. The histogram is used to represent the continuous data.

A histogram may look like the following graph:

The frequency polygon is a type of graphical representation which gives us better understanding of the shape of given distribution. Frequency polygons serve almost the similar purpose as histograms do. But the frequency polygon is quite helpful for the purpose of comparing two or more sets of data. The frequency polygons are said to be the extension of the histogram. When the midpoints of tops of the rectangular bars are joined together, the frequency polygon is made.

Few   examples    of    graphical    representation    of    statistical    data    are    given    below:

Example 1: Draw a dot plot for the following data.

Solution: The pie graph of the above data is:

Methods to Represent a Frequency Distribution:

Generally four methods are used to represent a frequency distribution graphically. These are Histogram, Smoothed frequency graph and Ogive or Cumulative frequency graph and pie diagram.

1. Histogram:

Histogram is a non-cumulative frequency graph, it is drawn on a natural scale in which the representative frequencies of the different class of values are represented through vertical rectangles drawn closed to each other. Measure of central tendency, mode can be easily determined with the help of this graph.

How to draw a Histogram:

Represent the class intervals of the variables along the X axis and their frequencies along the Y-axis on natural scale.

Start X axis with the lower limit of the lowest class interval. When the lower limit happens to be a distant score from the origin give a break in the X-axis n to indicate that the vertical axis has been moved in for convenience.

Now draw rectangular bars in parallel to Y axis above each of the class intervals with class units as base: The areas of rectangles must be proportional to the frequencies of the corresponding classes.

In this graph we shall take class intervals in the X axis and frequencies in the Y axis. Before plotting the graph we have to convert the class into their exact limits.

Advantages of histogram:

1.  It is easy to draw and simple to understand.

2.  It helps us to understand the distribution easily and quickly.

3.  It is more precise than the polygene.

Limitations of histogram:

1.  It is not possible to plot more than one distribution on same axes as histogram.

2.  Comparison of more than one frequency distribution on the same axes is not possible.

3.  It is not possible to make it smooth.

Uses of histogram:

1.Represents the data in graphic form.

2.Provides the knowledge of how the scores in the group are distributed. Whether the scores are piled up at the lower or higher end of the distribution or are evenly and regularly distributed throughout the scale.

3.Frequency Polygon. The frequency polygon is a frequency graph which is drawn by joining the coordinating points of the mid-values of the class intervals and their corresponding fre-quencies.

How to draw a frequency polygon:

Draw a horizontal line at the bottom of graph paper named ‘OX’ axis. Mark off the exact limits of the class intervals along this axis. It is better to start with i. of lowest value. When the lowest score in the distribution is a large number we cannot show it graphically if we start with the origin. Therefore put a break in the X axis to indicate that the vertical axis has been moved in for convenience. Two additional points may be added to the two extreme ends.

Draw a vertical line through the extreme end of the horizontal axis known as OY axis. Along this line mark off the units to represent the frequencies of the class intervals. The scale should be chosen in such a way that it will make the largest frequency (height) of the polygon approximately 75 percent of the width of the figure.

Plot the points at a height proportional to the frequencies directly above the point on the horizontal axis representing the mid-point of each class interval.

After plotting all the points on the graph join these points by a series of short straight lines to form the frequency polygon. In order to complete the figure two additional intervals at the high end and low end of the distribution should be included. The frequency of these two intervals will be zero.

Illustration: No. 7.3:

Draw a frequency polygon from the following data:

Advantages of frequency polygon:

2.  It is possible to plot two distributions at a time on same axes.

3.  Comparison of two distributions can be made through frequency polygon.

4.  It is possible to make it smooth.

Limitations of frequency polygon:

1.  It is less precise.

2.  It is not accurate in terms of area the frequency upon each interval.

Uses of frequency polygon:

1. When two or more distributions are to be compared the frequency polygon is used.

2. It represents the data in graphic form.

3. It provides knowledge of how the scores in one or more group are distributed. Whether the scores are piled up at the lower or higher end of the distribution or are evenly and regularly distributed throughout the scale.

2. Smoothed Frequency Polygon:

When the sample is very small and the frequency distribution is irregular the polygon is very jig-jag. In order to wipe out the irregularities and “also get a better notion of how the figure might  look if the data were more numerous, the frequency polygon may be smoothed.”

In this process to adjust the frequencies we take a series of ‘moving’ or ‘running’ averages. To get an adjusted or smoothed frequency we add the frequency of a class interval with the two adjacent intervals, just below and above the class interval. Then the sum is divided by 3. When these adjusted frequencies are plotted against the class intervals on a graph we get a smoothed frequency polygon.

Illustration 7.4:

Draw a smoothed frequency polygon, of the data given in the illustration No. 7.3:

Here we have to first convert the class intervals into their exact limits. Then we have to determine the adjusted or smoothed frequencies.

3. Ogive or Cumulative Frequency Polygon:

Ogive is a cumulative frequency graphs drawn on natural scale to determine the values of certain factors like median, Quartile, Percentile etc. In these graphs the exact limits of the class intervals  are shown along the X-axis and the cumulative frequencies are shown along the Y-axis. Below are given the steps to draw an ogive.

Get the cumulative frequency by adding the frequencies cumulatively, from the lower end (to get a less than ogive) or from the upper end (to get a more than ogive).

Mark off the class intervals in the X-axis.

Represent the cumulative frequencies along the Y-axis beginning with zero at the base.

Put dots at each of the coordinating points of the upper limit and the corresponding frequencies.

Join all the dots with a line drawing smoothly. This will result in curve called ogive.

Illustration No. 7.5:

Draw an ogive from the data given below:

To plot this graph first we have to convert, the class intervals into their exact limits. Then we have to calculate the cumulative frequencies of the distribution.

Uses of Ogive:

1.  Ogive is useful to determine the number of students below and above a particular score.

2.  When the median as a measure of central tendency is wanted.

3.  When the quartiles, deciles and percentiles are wanted.

4.  By plotting the scores of two groups on a same scale we can compare both the groups.

4. The Pie Diagram:

Figure given below shows the distribution of elementary pupils by their academic achievement in a school. Of the total, 60% are high achievers, 25% middle achievers and 15% low achievers. The construction of this pie diagram is quite simple. There are 360 degree in the circle. Hence, 60% of 360′ or 216° are counted off as shown in the diagram; this sector represents the proportion of high achievers students.

Ninety degrees counted off for the middle achiever students (25%) and 54 degrees for low achiever students (15%). The pie-diagram is useful when one wishes to picture proportions of the total in a striking way. Numbers of degrees may be measured off “by eye” or more accurately with a protractor.

Uses of Pie diagram:

1.  Pie diagram is useful when one wants to picture proportions of the total in a striking way.

2.  When a population is stratified and each strata is to be presented as a percentage at that time pie diagram is used.

PURPOSE OF GRAPHICAL REPRESENTATION:

The purpose of graphical presentation of data is to provide a quick and easy-to-read picture of information that clearly shows what otherwise takes a great deal of explanation. The impact of graphical data is typically more pointed and memorable than paragraphs of written information

For example, a person making a presentation regarding sales in various states across the country establishes the point of the presentation to the audience more quickly by using a color-coded map rather than merely stating the sales figures for each state. Observers quickly determine which states are ahead and which are behind in sales, and they know where emphasis needs to be placed. Alternatively, when making a presentation on sales by age groups using a pie chart that divides the pie into various ages, the audience quickly sees the results of sales by age. This  means that the audience is more likely to retain that information than if the presenter simply reads the results aloud or puts it into writing.

GENERAL RULES DISPLAYING DATA

• Simpler is Better
• Graphs, Tables and charts can be used together
• Use clear Description, title and labels
• Provide a narrative Description of the highlights
• Don’t compare variables with different scales of magnitude.
• A Diagram must be attractive, well proportioned,neat and pleasing to the eyes.
• They should be geometrically Accurate
• Size of the diagram should be proportional to paper should not be too big or too small
• Different colors should be used to classify data’s.

ADVANTAGES:

• Acceptability: graphical report is acceptable to the busy persons because it easily highlights about the theme of the report. This helps to avoid wastage of time.
• Comparative Analysis : Information can be compared in terms of graphical representation. Â Such comparative analysis helps for quick understanding and attention.
• Less cost : Information if descriptive involves huge time to present properly. It involves more money to print the information but graphical presentation can be made in short but catchy view to make the report understandable. It obviously involves less cost.
• Decision Making: Business executives can view the graphs at a glance and can make decision very quickly which is hardly possible through descriptive report.
• Logical Ideas: If tables, design and graphs are used to represent information then a logical sequence is created to clear the idea of the audience.
• Helpful for less literate Audience: Less literate or illiterate people can understand graphical representation easily because it does not involve going through line by line of any descriptive report.
• Less Effort and Time: To present any table, design, image or graphs require less effort and time. Furthermore, such presentation makes quick understanding of the information.
• Less Error and Mistakes: Qualitative or informative or descriptive reports involve errors or mistakes. As graphical representations are exhibited through numerical figures, tables or graphs, it usually involves less error and mistake.
• A complete Idea: Such representation creates clear and complete idea in the mind of audience. Reading hundred pages may not give any scope to make decision. But an instant view or looking at a glance obviously makes an impression in  the mind of audience regarding the topic or subject.
• Use in the Notice Board: Such representation can be hanged in the notice board to quickly raise the attention of employees in any organization.

DISADVANTAGES:

Graphical representation of reports is not free from limitations. The following are the problems of graphical representation of data or reports:

• Costly : Graphical representation pf reports are costly because it involves images, colors and paints. Combination of material with human efforts makes the graphical presentation expensive.
• More time : Normal report involves less time to represent but graphical representation involves more time as it requires graphs and figures which are dependent to more time.
• Errors and Mistakes : Since graphical representations are complex, there is- each and every chance of errors and mistake. This causes problems for better understanding to general people.
• Lack of Secrecy: Graphical representation makes full presentation of information which may hamper the objective to keep something secret.
• Problems to select the suitable method: Information can be presented through various graphical methods and ways. Which should be the suitable method is very hard to select.
• Problem of Understanding: All may not be able to get the meaning of graphical representation because it involves various technical matters which are complex to general people.

Last of all it can be said that graphical representation does not provide proper information to general people.

CONCLUSION:

Graphical representation makes the datamore possible to easily draw; visual impression of data. Graphical representation of data enhances the understandings of the observer. It makes comparisons easy. This kind of method creates an imprint on mind for a long period of time. Well in this chapter we have discussed about the definition ,types ,advantages and disadvantages in detail with relevant examples which will have an impact in the power of understanding. I request you all to go through the various types of graphs commonly used in research studies in with reference to home science research studies to explore new ideas in the field of research.

• http://shodhganga.inflibnet.ac.in/bitstream/10603/143688/2/file%202%20chapter%201 %20data%20representation%20techniques.pdf
• http://www.mas.ncl.ac.uk/~ndah6/teaching/MAS1403/notes_chapter2.pdf https://www.ncbi.nlm.nih.gov/pmc/articles/PMC5453888/
• http://cec.nic.in/wpresources/module/Anthropology/PaperIX/9/content/downloads/file1. pdf
• https://www.kluniversity.in/arp/uploads/2096.pdf

Talk to our experts

1800-120-456-456

• Graphs and Graphical Representation

What are Graphs and Graphical Representation?

Graphical representation refers to the use of charts and graphs to visually analyze and display, interpret numerical value, clarify the qualitative structures. The data is represented by a variety of symbols such as line charts, bars, circles, ratios. Through this, greater insight is stuck in the mind while analyzing the information. 

Graphs can easily illustrate the behavior, highlight changes, and can study data points that may sometimes be overlooked. The type of data presentation depends upon the type of data being used. 

Graphical Representation of Data

The graphical representation is simply a way of analyzing numerical data. It comprises a relation between data, information, and ideas in a diagram. Anything portrayed in a graphical manner is easy to understand and is also termed as the most important learning technique. The graphical presentation is always dependent on the type of information conveyed. There are different types of graphical representation. These are as follows:

Line Graphs: 

Also denoted as linear graphs are used to examine continuous data and are also useful in predicting future events in time.

Histograms: 

This graph uses bars to represent the information. The bars represent the frequency of numerical data. All intervals are equal and hence, the width of each bar is also equal.

Bar Graphs: 

These are used to display the categories and compare the data using solid bars. These bars represent the quantities.

Frequency Table: 

This table shows the frequency of data that falls within that given time interval. 

Line Plot: 

It shows the frequency of data on a given line number.

Circle Graph: 

It is also known as a pie chart and shows the relationship between the parts of the whole. The circle consists of 100% and other parts shown are in different proportions.

Scatter Plot: 

The diagram shows the relationship between two sets of data. Each dot represents individual information of the data.

Venn Diagram: 

It consists of overlapping circles, each depicting a set. The inner-circle made is a graphical representation.

Stem and Leaf Plot: 

The data is organized from the least value to the highest value. The digits of the least place value form the leaf and that of the highest place value form the stem.

 Box and Whisker Plot: 

The data is summarised by dividing it into four parts. Box and whisker show the spread and median of the data.

Graphical Presentation of Data - Definition

It is a way of analyzing numerical data. It is a sort of chart which shows statistical data in the form of lines or curves which are plotted on the surface. It enables studying the cause and effect relationships between two variables . It helps to measure the extent of change in one variable when another variable changes. 

Principles of Graphical Representation

The variables in the graph are represented using two lines called coordinate axes. The horizontal and vertical axes are denoted by x and y respectively. Their point of intersection is called an origin ‘O’. Considering x-axes, the distance from the origin to the right will take a positive value, and the distance from the origin to the left will take a negative value. Taking the same procedure on y-axes. The points above origin will take the positive values and the points below origin will take negative values. As discussed in the earlier section about the types of graphical representation. There are four most widely used graphs namely histogram, pie diagram, frequency polygon, and ogive frequency graph.

Rules for Graphical Representation of Data

There are certain rules to effectively represent the information in graphical form. Certain rules are discussed below:

Title: One has to make sure that a suitable title is given to the graph which indicates the presentation subject.

Scale: It should be used efficiently to represent data in an accurate manner.

Measurement unit: It is used to calculate the distance between the box

Index: Differentiate appropriate colors, shades, and design I graph for a better understanding of the information conveyed.

Data sources: Include the source of information at the bottom graph wherever necessary. It adds to the authenticity of the information. 

Keep it simple: Construct the graph in an easy to understand manner and keep it simple for the reader to understand. Looking at the graph the information portrayed is easily understandable. 

Importance of Graphical Representation of Data

Some of the importance and advantages of using graphs to interpret data are listed below:

The graph is easiest to understand as the information portrayed is in facts and figures. Any information depicted in facts, figures, comparison grabs our attention, due to which they are memorizable for the long term.

It allows us to relate and compare data for different time periods.

It is used in statistics to determine the mean , mode, and median of different data.

It saves a lot of time as it covers most of the information in facts and figures. This in turn compacts the information.

FAQs on Graphs and Graphical Representation

Q1. State the Advantages and Disadvantages of Graphical Representation of Data?

Ans: These graphical presentations of data are vital components in analyzing the information. Data visualization is one of the most fundamental approaches to data representation. Its advantages include the following points:

Facilitates and improves learning

Flexibility of use

Understands content

Increase structure thinking

Supports creative thinking

Portrays the whole picture

Improves communication

With advantages, certain disadvantages are also linked to the graphical representation. The disadvantages concern the high cost of human effort, the process of selecting the most appropriate graphical and tabular presentation, creative thinking, greater design to interpret information, visualizing data, and as human resource is used. The potential for human bias plays a huge role.

Q2. What is the Graphical Representation of Data in Statistics?

Graphs are powerful data evaluation tools. They provide a quick visual summary of the information. In statistics information depicted is of mean, mode, and median. Box plots, histograms are used to depict the information. These graphs provide information about ranges, shapes, concentration, extreme values, etc. It studies information between different sets and trends whether increasing or decreasing. Since graphical methods are qualitative, they are not only the basis of comparison and information.

Introduction to Graphs

Table of Contents

15 December 2020                 

Read time: 6 minutes

Introduction

What are graphs?

What are the different types of data?

What are the different types of graphical representations?

The graph is nothing but an organized representation of data. It helps us to understand the data. Data are the numerical information collected through observation.

The word data came from the Latin word Datum which means “something given”

After a research question is developed, data is being collected continuously through observation. Then it is organized, summarized, classified, and then represented graphically.

Differences between Data and information: Data is the raw fact without any add on but the information is the meaning derived from data.

Introduction to Graphs-PDF

The graph is nothing but an organized representation of data. It helps us to understand the data. Data are the numerical information collected through observation. Here is a downloadable PDF to explore more.

• Line and Bar Graphs Application
• Graphs in Mathematics & Statistics

What are the different Types of Data?

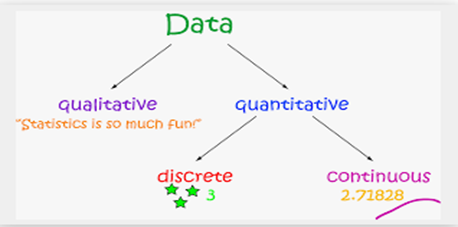
There are two types of Data :

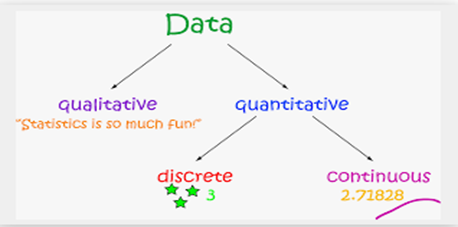
Quantitative

The data which are statistical or numerical are known as Quantitive data. Quantitive data is generated through. Quantitative data is also known as Structured data. Experiments, Tests, Surveys, Market Report.

Quantitive data is again divided into Continuous data and Discrete data.

Continuous Data

Continuous data is the data which can have any value. That means Continuous data can give infinite outcomes so it should be grouped before representing on a graph.

• The speed of a vehicle as it passes a checkpoint
• The mass of a cooking apple
• The time taken by a volunteer to perform a task

Discrete Data

Discrete data can have certain values. That means only a finite number can be categorized as discrete data.

• Numbers of cars sold at a dealership during a given month
• Number of houses in certain block
• Number of fish caught on a fishing trip
• Number of complaints received at the office of airline on a given day
• Number of customers who visit at bank during any given hour
• Number of heads obtained in three tosses of a coin

Differences between Discrete and Continuous data

• Numerical data could be either discrete or continuous
• Continuous data can take any numerical value (within a range); For example, weight, height, etc.
• There can be an infinite number of possible values in continuous data
• Discrete data can take only certain values by finite ‘jumps’, i.e., it ‘jumps’ from one value to another but does not take any intermediate value between them (For example, number of students in the class)

Qualitative

Data that deals with description or quality instead of numbers are known as Quantitative data. Qualitative data is also known as unstructured data. Because this type of data is loosely compact and can’t be analyzed conventionally.

Different Types of Graphical Representations

There are many types of graph we can use to represent data. They are as follows,

A bar graph or chart is a way to represent data by rectangular column or bar. The heights or length of the bar is proportional to the values.

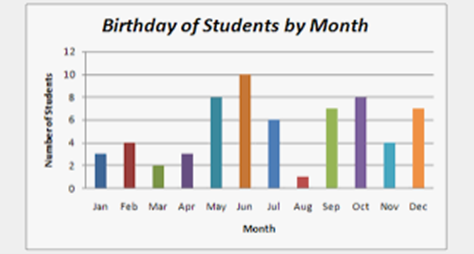
A line graph is a type of graph where the information or data is plotted as some dots which are known as markers and then they are added to each other by a straight line.

The line graph is normally used to represent the data that changes over time.

A histogram graph is a graph where the information is represented along with the height of the rectangular bar. Though it does look like a bar graph, there is a fundamental difference between them. With the histogram, each column represents a range of quantitative data when a bar graph represents categorical variables.

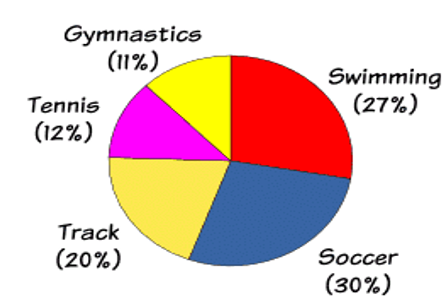
The other name of the pie chart is a circle graph. It is a circular chart where numerical information represents as slices or in fractional form or percentage where the whole circle is 100%.

• Stem and leaf plot

The stem and leaf plot is a way to represents quantitative data according to frequency ranges or frequency distribution.

In the stem and leaf plot, each data is split into stem and leaf, which is 32 will be split into 3 stems and 2 leaves.

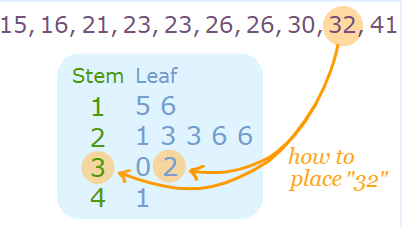
Frequency table: Frequency means the number of occurrences of an event. A frequency distribution table is a graph or chart which shows the frequency of events. It is denoted as ‘f’ .

Pictograph or Pictogram is the earliest way to represents data in a pictorial form or by using symbols or images. And each image represents a particular number of things.

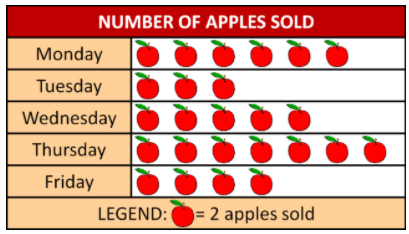
According to the above-mentioned Pictograph, the number of Appels sold on Monday is 6x2=12.

• Scatter diagrams

Scatter diagram or scatter plot is a way of graphical representation by using cartesian coordinates of two variables. The plot shows the relationship between two variables. Below there is a data table as well as a Scattergram as per the given data.

What is the meaning of Graphical representation?

Graphical representation is a way to represent and analyze quantitive data. A graph is a kind of a chart where data are plotted as variables across the coordinate. It became easy to analyze the extent of change of one variable based on the change of other variables.

Principles of graphical representation

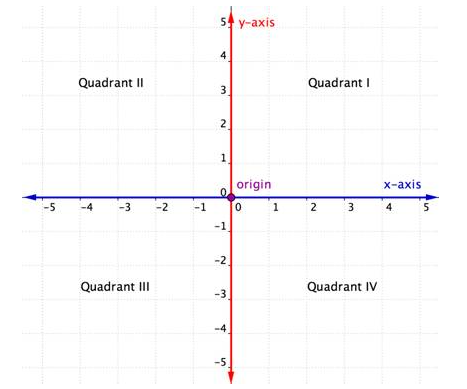
The principles of graphical representation are algebraic. In a graph, there are two lines known as Axis or Coordinate axis. These are the X-axis and Y-axis. The horizontal axis is the X-axis and the vertical axis is the Y-axis. They are perpendicular to each other and intersect at O or point of Origin.

On the right side of the Origin, the Xaxis has a positive value and on the left side, it has a negative value. In the same way, the upper side of the Origin Y-axis has a positive value where the down one is with a negative value.

When X-axis and y-axis intersected each other at the origin it divides the plane into four parts which are called Quadrant I, Quadrant II, Quadrant III, Quadrant IV.

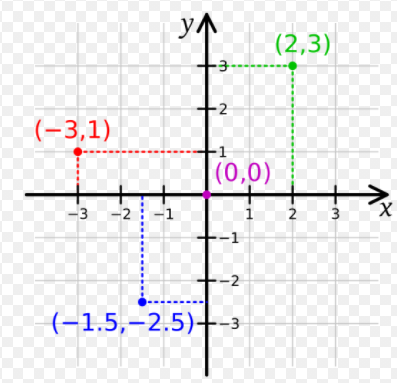
The location on the coordinate plane is known as the ordered pair and it is written as (x,y). That means the first value will be on the x-axis and the second one is on the y-axis. When we will plot any coordinate, we always have to start counting from the origin and have to move along the x-axis, if it is positive then to the right side, and if it is negative then to the left side. Then from the x-axis, we have to plot the y’s value, which means we have to move up for positive value or down if the value is negative along with the y-axis.

In the following graph, 1st ordered pair (2,3) where both the values of x and y are positive and it is on quadrant I. 2nd ordered pair (-3,1), here the value of x is negative and value of y is positive and it is in quadrant II. 3rd ordered pair (-1.5, -2.5), here the value of x as well as y both are Negative and in quadrant III.

Methods of representing a frequency distribution

There are four methods to represent a frequency distribution graphically. These are,

• Smoothed Frequency graph
• Cumulative frequency graph or Ogive.
• Pie diagram.

Advantages and Disadvantages of Graphical representation of data

• It improves the way of analyzing and learning as the graphical representation makes the data easy to understand.
• It can be used in almost all fields from mathematics to physics to psychology and so on.
• It is easy to understand for its visual impacts.
• It shows the whole and huge data in an instance.

The main disadvantage of graphical representation of data is that it takes a lot of effort as well as resources to find the most appropriate data and then represents it graphically.

You may also like:

• Graphing a Quadratic Function
• Empirical Relationship Between Mean, Median, and Mode

Not only in mathematics but almost in every field the graph is a very important way to store, analyze, and represents information. After any research work or after any survey the next step is to organize the observation or information and plotting them on a graph paper or plane. The visual representation of information makes the understanding of crucial components or trends easier.

A huge amount of data can be store or analyze in a small space.

The graphical representation of data helps to decide by following the trend.

A complete Idea: Graphical representation constitutes a clear and comprehensive idea in the minds of the audience. Reading a large number (say hundreds) of pages may not help to make a decision. Anyone can get a clear idea just by looking into the graph or design.

Graphs are a very conceptual topic, so it is essential to get a complete understanding of the concept. Graphs are great visual aids and help explain numerous things better, they are important in everyday life. Get better at graphs with us, sign up for a free trial . 

About Cuemath

Cuemath, a student-friendly mathematics and coding platform, conducts regular Online Classes for academics and skill-development, and their Mental Math App, on both iOS and Android , is a one-stop solution for kids to develop multiple skills. Understand the Cuemath Fee structure and sign up for a free trial.

Frequently Asked Questions (FAQs)

What is data.

Data are characteristics or information, usually numerical, that are collected through observation.

How do you differentiate between data and information?

Data is the raw fact without any add on but the information is the meaning derived from data.

What are the types of data?

There are two types of Data:

What are the ways to represent data?

Tables, charts and graphs are all ways of representing data , and they can be used for two broad purposes. The first is to support the collection, organisation and analysis of data as part of the process of a scientific study.

- Tables, charts and graphs are all ways of representing data, and they can be used for two broad purposes. The first is to support the collection, organisation and analysis of data as part of the process of a scientific study.

What are the different types of graphs?

Different types of graphs include: